This is a revisit of a subject that I first broached in this post, which I felt needed more examination. So let’s return to that radical concept of Phi.
Phi is a mathematical ratio that, curiously enough, has more than a few close representations in nature. It is an irrational number, one of those decimals that go on forever, but usually shortened to 1.618. If you create a rectangle with dimensions that follow this ratio, what it means is the long sides will be 1.618 times as long as the short side. If you were to subdivide the same ratio within that rectangle – turning the short sides into the long sides of another rectangle that sits inside the first – you can produce an ever-decreasing set of shapes within, turning 90° at a time; alternately you can expand by the same amount. If you then sketch a curve within each rectangle between opposing corners (90° arc,) you produce an expanding spiral like a nautilus shell. You can go here for a basic, and level-headed, mathematical visualization, and here for a great example of how to read way the hell too much into the idea.
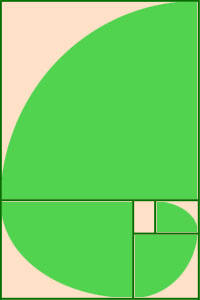 Why do I say that? Well, it’s simple. Try the same thing with the number 1.5 instead of 1.618. It would look like this. You can, in fact, use any ratio that you want and produce both a spiral and a nice descending composition, because that’s the nature of ratios – this isn’t anything profound. Phi is just neat: A/B=B/C.
Why do I say that? Well, it’s simple. Try the same thing with the number 1.5 instead of 1.618. It would look like this. You can, in fact, use any ratio that you want and produce both a spiral and a nice descending composition, because that’s the nature of ratios – this isn’t anything profound. Phi is just neat: A/B=B/C.
Now we come to where it starts going silly. Using the first link I provided, you’ll notice that their diagram of the rectangle is claimed to look, “like a typical frame for a painting.” Well, yes, kind of, but the same can be said for many rectangles, and if we’re going to be specific, let’s take a look at the common photographic print sizes. 4×6 is a ratio of 1.5, 5×7 is 1.4, 8×10 is 1.25, and 11×14 is 1.27. Huh. Okay, but how about monitor and TV screen sizes, or widescreen ratios? Nope – 1.33 to 1.77, typically. If the claims are that a ratio of 1.618 is “most pleasing,” then why are we incapable of using it?
Okay, but wait. The same page shows how well the Parthenon fits the ratio, nicely diagrammed. Check it out (I’m not going to embed the images without permission, and not going to even try to obtain it just for this post.) Looks good? Unless, of course, you consider that two of their examples are measuring to the peaked roof, which seems to me to be fudging things a bit – does a triangle in that ratio count? Diagram a triangle either way and see if it seems “most pleasing.” But also, take a look at the most distinctive, and immediately apparent, rectangle of the Parthenon’s façade, the row of columns in the front. Whoops, nobody diagrammed that, and with good reason, since it doesn’t fit the ratio. Even including the header, it doesn’t come close. And this was the reasonable page.
You can go way off the mark if you go to that other page, and believe me, this is far from the only example online. I hate linking to such things because they really don’t need the traffic. You can diagram a Phi spiral onto a human ear, for example – as long as you find the right ear. 1.618 seconds of thought will tell you that not every ear looks the same, and this can be said for every other example where ‘nature’ demonstrates this remarkable mathematical property. Most snail and nautilus shells do not follow this ratio closely, since they depend on just how fast the critter is growing; nor do most seed spirals from sunflowers peg it, and if we stop using the examples that seem to match and just do a cross section of any flower head, nature’s wonderful reliance suddenly produces an abysmal hit rate.
 Now, I’ll be fair. Sometimes, there are some pretty close fits to mathematical ratios in nature, but this shouldn’t be surprising in the least. Organisms that produce the most efficient ‘designs’ obviously will thrive better than those with less efficiency, so the trend will be towards maximum efficiency. But the keyword there is “trend,” and obtaining an average from a cross-sampling of species can only provide useful information in specific circumstances. Using an image of a real nautilus shell (Wikipedia Commons,) we really cannot see the ratio at work at all – now you know why Phi is illustrated by drawings so often. The overall ratio (outside rectangle) is 1.28, while the growth ratios wander about a bit. I scribed lines every 90° to roughly ‘center,’ which didn’t quite work, but also measured from center, getting growth rates from 1.23 to 1.43, which is a far cry from ‘logarithmic,’ especially when they went back and forth! Basically, what we’re dealing with here is a ridiculous attempt to force a fit rather than finding something either explanatory or ‘typical.’
Now, I’ll be fair. Sometimes, there are some pretty close fits to mathematical ratios in nature, but this shouldn’t be surprising in the least. Organisms that produce the most efficient ‘designs’ obviously will thrive better than those with less efficiency, so the trend will be towards maximum efficiency. But the keyword there is “trend,” and obtaining an average from a cross-sampling of species can only provide useful information in specific circumstances. Using an image of a real nautilus shell (Wikipedia Commons,) we really cannot see the ratio at work at all – now you know why Phi is illustrated by drawings so often. The overall ratio (outside rectangle) is 1.28, while the growth ratios wander about a bit. I scribed lines every 90° to roughly ‘center,’ which didn’t quite work, but also measured from center, getting growth rates from 1.23 to 1.43, which is a far cry from ‘logarithmic,’ especially when they went back and forth! Basically, what we’re dealing with here is a ridiculous attempt to force a fit rather than finding something either explanatory or ‘typical.’
The same can be said, shouted really, with all of the other examples of Phi in ‘great art,’ and literature, and so on. First off, there’s the basic nature of numerology. Pick any number or ratio, and go out looking for all of the places where it can be fitted in someplace. Found a bunch? Yeah, this is hardly stunning, especially if you don’t mind fudging the fit a bit – you can make a ratio of any point A to any point B that you like, and then go find matches. This is where a basic understanding of science comes in helpful, because the idea of looking for exceptions is important. You can see a lot of 1.618 ratios? Good, now try 1.5, and 1.75, and so on. If you find far fewer of these than 1.618, you might have a reason to be impressed.
But even this wouldn’t be very informative, since artwork and literature are influenced by cultures and biases. Did some of the classic artists use the ratio in their works? It’s quite possible, even though the numbers aren’t too convincing when someone starts looking for exceptions rather than fits. But such reliance only represents the quirks of the artist, perhaps their belief that it was important. If, for instance, changing the ratio to something else would completely ruin the artistic merit of the work, then perhaps a case could be made for Phi being important. But just because any popular work reflects some reliance on an idea doesn’t mean it’s the only thing that could have worked, or that the artist was onto some big secret. If you like arbitrary rules, you could notice that most great works of art feature humans, even though we are just one of millions of species on the planet – is this profound? Does this make humans special? Well, if by “special” you only mean “egocentric,” then yes. We’re special as hell.
Then there’s the unanswered question in the middle of all this, which is, “If Phi is indicative of something, what is it indicative of?” Why is some mathematical property supposed to be something special? Math is simply ratios to begin with – it is entirely abstract, and requires arbitrary definitions to even be able to apply to anything physical. Even if we were to find some very specific ratio in nature that is not explained in “Well, duh!” terms, like exponential growth or size/mass comparisons, what would this mean? Some people, perhaps a lot, take it to mean that there is a grand design, as if mathematical ratios could not spring up naturally.
What this really demonstrates, however, is how easily we come at something from the wrong side, making assumptions or succumbing to internal bias. We are pattern-seeking animals; we get a positive reaction from finding something that seems to fit some kind of formula, whether it be visual symmetry, or trends in growth times, or just number patterns. It can be useful to us, because it lets us discern the camouflaged animal from random foliage, or create calendars to judge planting times, or see where monetary trends may eventually lead. But it’s imperfect in a certain regard, in that we get little to no positive reaction from discovering a lack of patterns, or a misleading situation, so very often we don’t even try.
This is the nature of critical thought, and something that we need more often than we might even think. We’re actually biased towards finding patterns, and truth be told, it can be very easy to find them. They mean nothing, however, unless they’re more prevalent than the lack thereof, or demonstrate something useful. We need to provoke ourselves to look for the lack every time we think we’ve found a pattern, just to determine if the pattern really exists – without this cognitive decision, we’re only responding to primitive internal goads rather than discovering useful information.




















































